This hypothesis requires the lack of Earth-sized planets (compared to planets twice as large) to be real, and not a result of poorer detection efficiency for Earth-sized planets relative to slightly larger ones.
Commenter number one suggested that my figure two was not a convincing demonstration of this, and a comparison of planet size vs. spectral type (a proxy for effective temperature) or stellar radius would be more convincing. So here are those plots.
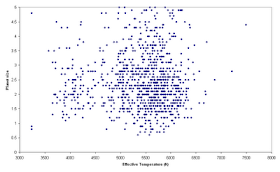
Figure 1. Planetary radius (relative to Earth) vs. effective temperature.

Figure 2. Planetary radius (relative to Earth) vs. stellar radius (relative to the Sun).
Figure 1 shows that earth-sized detections decrease for stars hotter than about 6000K, and figure 2 shows that they decrease for stars with a radius of more than 1.2 solar radii. This is not surprising, as the hotter stars will generally have larger radii, so a planet of a given size will block proportionally less light.
To correct for this, I have replotted a subset of the data release. Figure 3 is a probability distribution curve consists of only stars which have radii less than 1.1 times that of the Sun.

Figure 3. Probability distribution curve of planets around stars with stellar radii less than 1.1 times that of the sun.
Filtering out the large radii stars leaves a sample size of 953. The general shape of the curve is not too different than the whole sample. So the hypothesis that the most common size of a planet is midway between Earth and Neptune still seems to hold.
As I commented elsewhere (just joking, but it was on Cosmic Variance) the real statistics is imprecise given how we find all systems different so far (many possible outcomes) and there seems to be many ways to make them (many future models).
ReplyDeleteHowever the largest problem here is that you don't offer the probability of picking our SS out of your distribution. It may not actually be rare if we run, say, Monte Carlos of systems building to the distribution in question.
Then most systems will have one planet, some will have two, et cetera; by the time you get to 8 planets presumably they will all be odd balls even without any actual formation pathway information on how systems are build. All odd balls = SS not rare.